Sin3x differentiation
Derivative of sin3x is 3cos3x. It is part of Differentiation which is a sub-topic of calculus. Sin3x sin3x differentiation a composite function of two elementary functions namely, algebraic function and trigonometric function. In the derivative of sin3x, 3x is a pure algebraic function whereas sin[f x ] is a trigonometric function, sin3x differentiation.
The derivative of sin3x is equal to 3cos3x. We can evaluate the differentiation of sin3x using different methods of derivatives such as the first principle of derivatives and the chain rule method. We will also determine the formula for the derivative of sin3x using the first principle and the formula for the derivative of sin cube x and solve some examples related to the concept for a better understanding of the concept. Differentiation of sin3x is the process of finding its derivative which can be determined using various differentiation methods. We can find the derivative of sin3x using the first principle of derivatives, that is, the definition of limits and the chain rule method of differentiation. In the next section, let us explore the formula for the derivative of sin3x. The image given below shows the formula of sin3x differentiation:.
Sin3x differentiation
The chain rule is a tool for differentiating composite functions, that is, a function inside a function. Here, we have sin 3x. This can be thought of as the function 3x being put inside of the function sin x. When finding the derivative of such a function, the chain rule tells us that the derivative will be equal to the derivative of the outside function with the original inside function still inside of it, all multiplied by the derivative of the inside function. So, for sin 3x , the derivative the sin x , the outside function, is cos x. So, the first part of the chain rule, the differentiated outside function with the inside function unchanged, gives us cos 3x. Then, this is multiplied by the derivative of the inside function. What is the derivative of sin 3x? Jun 14, Explanation: The chain rule is a tool for differentiating composite functions, that is, a function inside a function.
Example 3: Find the derivative of sin3x cos3x. Example 4: What is the nth derivative of sin 2x cos 3x.
Note that in this post we will be looking at differentiating sin 3x which is not the same as differentiating sin 3 x. Here is our post dealing with how to differentiate sin 3 x. The chain rule is useful for finding the derivative of a function which could have been differentiated had it been in x, but it is in the form of another expression which could also be differentiated if it stood on its own. To perform the differentiation sin 3x , the chain rule says we must differentiate the expression as if it were just in terms of x as long as we then multiply that result by the derivative of what the expression is actually in terms of in this case the derivative of 3x. The Chain Rule: For two differentiable functions f x and g x. Now we can just plug f x and g x into the chain rule. But before we do that, just a quick recap on the derivative of the sin function.
The derivative of sin3x is equal to 3cos3x. We can evaluate the differentiation of sin3x using different methods of derivatives such as the first principle of derivatives and the chain rule method. We will also determine the formula for the derivative of sin3x using the first principle and the formula for the derivative of sin cube x and solve some examples related to the concept for a better understanding of the concept. Differentiation of sin3x is the process of finding its derivative which can be determined using various differentiation methods. We can find the derivative of sin3x using the first principle of derivatives, that is, the definition of limits and the chain rule method of differentiation. In the next section, let us explore the formula for the derivative of sin3x. The image given below shows the formula of sin3x differentiation:.
Sin3x differentiation
One of the most important types of motion in physics is simple harmonic motion, which is associated with such systems as an object with mass oscillating on a spring. Simple harmonic motion can be described by using either sine or cosine functions. In this section we expand our knowledge of derivative formulas to include derivatives of these and other trigonometric functions. We begin with the derivatives of the sine and cosine functions and then use them to obtain formulas for the derivatives of the remaining four trigonometric functions. Being able to calculate the derivatives of the sine and cosine functions will enable us to find the velocity and acceleration of simple harmonic motion. We begin our exploration of the derivative for the sine function by using the formula to make a reasonable guess at its derivative. Indeed, we will show that. If we were to follow the same steps to approximate the derivative of the cosine function, we would find that. The derivative of the sine function is the cosine and the derivative of the cosine function is the negative sine.
Torn crossword clue
Stay tuned to the Testbook App for more updates on related topics from Mathematics, and various such subjects. The derivatives of these two functions can be calculated separately as given below: Derivative of 3x: The derivative of a variable with respect to the same variable is equal to one. The first derivative of sin3x is equal to 3 cos3x. Differentiation of sin3x is the process of finding its derivative which can be determined using various differentiation methods. Commercial Maths. Skip to content The derivative of sin 3x is 3cos 3x How to calculate the derivative of sin 3x Note that in this post we will be looking at differentiating sin 3x which is not the same as differentiating sin 3 x. Is sin 3x even or odd? Also, reach out to the test series available to examine your knowledge regarding several exams. We hope that the above article is helpful for your understanding and exam preparations. When finding the derivative of such a function, the chain rule tells us that the derivative will be equal to the derivative of the outside function with the original inside function still inside of it, all multiplied by the derivative of the inside function.
The chain rule is a tool for differentiating composite functions, that is, a function inside a function. Here, we have sin 3x.
Explanation: The chain rule is a tool for differentiating composite functions, that is, a function inside a function. It is useful when finding the derivative of a function that is raised to the nth power. Maths Program. The derivative of sin x with respect to x is cos x The derivative of sin z with respect to z is cos z In a similar way, the derivative of sin 3x with respect to 3x is cos 3x. In the derivative of sin3x, 3x is a pure algebraic function whereas sin[f x ] is a trigonometric function. Math worksheets and visual curriculum. The derivative is a measure of the instantaneous rate of change, which is equal to:. Commercial Maths. Privacy Policy. Multiplication Tables. So, for sin 3x , the derivative the sin x , the outside function, is cos x.

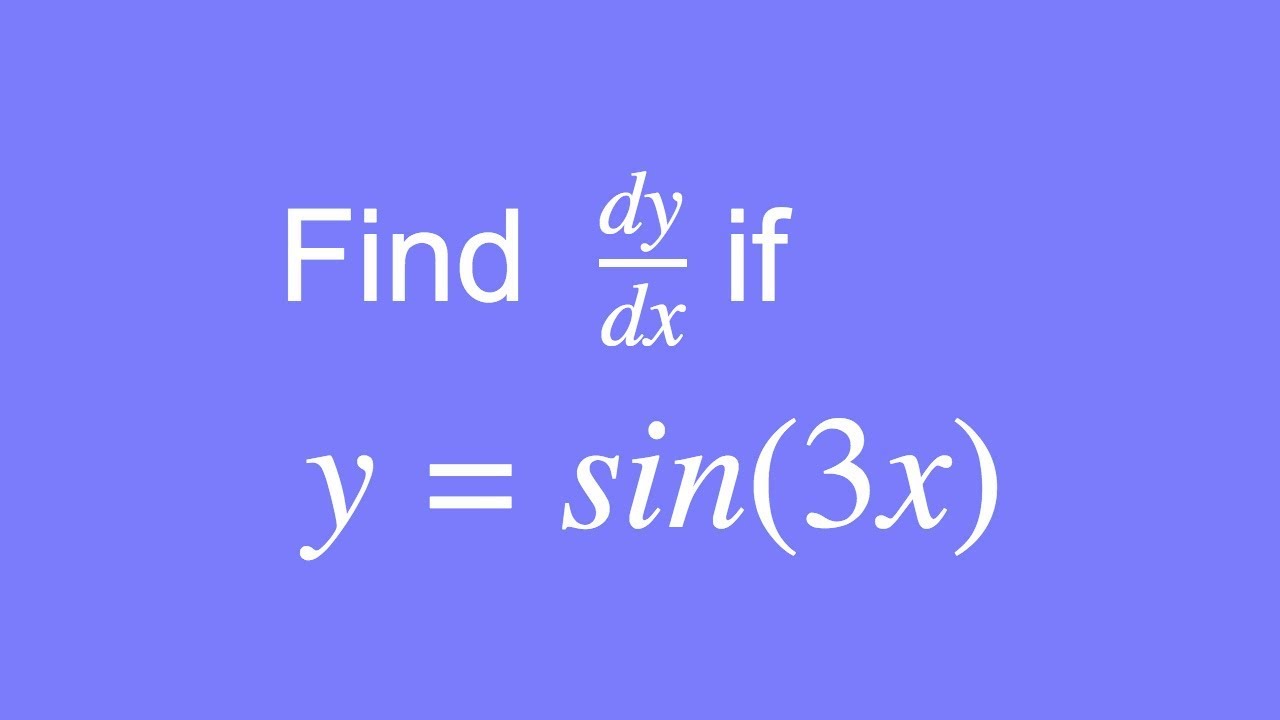
0 thoughts on “Sin3x differentiation”