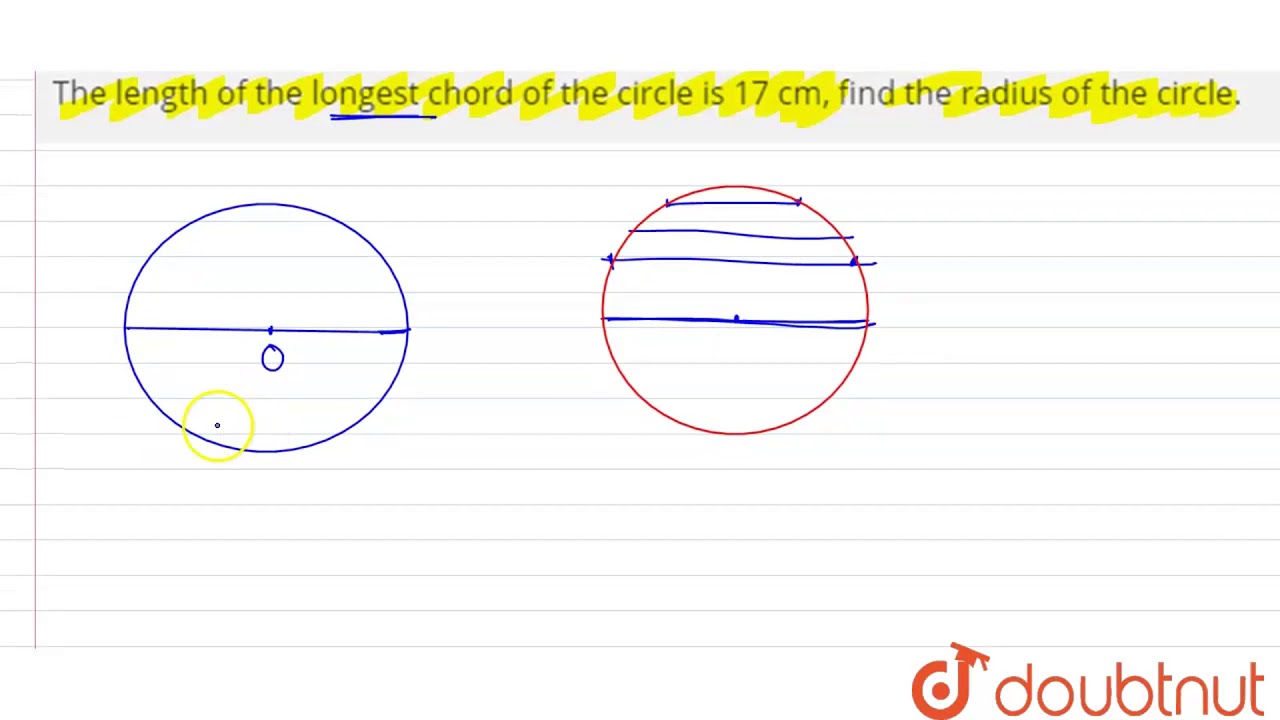
The longest chord of the circle

Geometry is the major part of mathematics that deals with lines, angles, points, etc. They are the visual study of shapes and sizes. The geometric approach is seen everywhere around us as every object has a certain shape whose parameters can be studied with the help of geometrical formulas.

Indeed why? Why a diameter is the longest chord in a circle? I sometimes heard and several times read at popular math sites that the reason why a diameter is the longest chord is that a diameter passes through the center of the circle. While that is true that passing through the center has something to do with the length of a chord, the answer, as given, is vacuous. Since the definition of a diameter is a chord passing through the center of the circle, such an explanation actually reads: "The diameter is the longest chord in a circle because it is a diameter of the circle.

The longest chord of the circle

.

You will be notified via email once the article is available for improvement. Open In App.

.

The chord of a circle is defined as the line segment joining any two points on the circumference of the circle. It should be noted that the diameter is the longest chord of a circle that passes through the center of the circle. A line segment that joins two points on the circumference of the circle is defined as the chord of the circle. Among the other line segments that can be drawn in a circle, the chord is one whose endpoints lie on the circumference. Observe the following circle to identify the chord PQ. Diameter is also considered to be a chord which passes through the center of the circle. Theorem 1: The perpendicular to a chord, drawn from the center of the circle, bisects the chord. Observe the following circle to understand the theorem in which OP is the perpendicular bisector of chord AB and the chord gets bisected into AP and PB. Theorem 2: Chords of a circle, equidistant from the center of the circle are equal. Theorem 3: For two unequal chords of a circle, the larger chord will be closer to the center than the smaller chord.

The longest chord of the circle

The chord of a circle can be defined as the line segment joining any two points on the circumference of the circle. It should be noted that the diameter is the longest chord of a circle which passes through the center of the circle. The figure below depicts a circle and its chord. Let us consider the chord CD of the circle and two points P and Q anywhere on the circumference of the circle except the chord as shown in the figure below. Question: Find the length of the chord of a circle where the radius is 7 cm and perpendicular distance from the chord to the center is 4 cm. If we try to establish a relationship between different chords and the angle subtended by them in the center of the circle, we see that the longer chord subtends a greater angle at the center.

Bayside council clean up dates 2023

Please Login to comment Improved By :. What is the longest chord of a Circle? Incidentally, Euclid proved that statement in the third book of his Elements as a more informative Proposition XV : Of straight lines in a circle the diameter is greatest, and of the rest the nearer to the center is always greater than the more remote. While that is true that passing through the center has something to do with the length of a chord, the answer, as given, is vacuous. Theorem - The lengths of tangents drawn from an external point to a circle are equal - Circles Class 10 Maths. Why Diameter Is the Longest Chord? Question 4: Determine the diameter of a circle with a radius of 16cm. Create Improvement. On a casual inspection, it seems obvious: Nonetheless, it has to be proved and Euclid proves the first part with the reference to the Triangle Inequality I. Theorem - The tangent at any point of a circle is perpendicular to the radius through the point of contact - Circles Class 10 Maths. The longest chord in a circle passes through the center of the circle and is, therefore, a diameter. As the statement is definitely not an axiom of geometry, it must be proved , i. The longest chord of a circle is its diameter, which is double the radius of the circle.

A chord from the Latin chorda , meaning " bowstring " of a circle is a straight line segment whose endpoints both lie on a circular arc.

This article is being improved by another user right now. Nonetheless, it has to be proved and Euclid proves the first part with the reference to the Triangle Inequality I. Change Language. Admission Experiences. Question 1: Determine the diameter of a circle with a radius of 8cm. Article Tags :. Incidentally, Euclid proved that statement in the third book of his Elements as a more informative Proposition XV : Of straight lines in a circle the diameter is greatest, and of the rest the nearer to the center is always greater than the more remote. Share your suggestions to enhance the article. Thank you for your valuable feedback! Theorem - There is one and only one circle passing through three given non-collinear points Class 9 Maths. Hire With Us.