Rhs similarity criterion

Two triangles are said to be congruent to each other if the measurements of their three sides and their three angles are exactly the same. They may be rotated or flipped, rhs similarity criterion.

Measurement and Geometry : Module 22 Years : PDF Version of module. Scale drawings are used when we increase or reduce the size of an object so that it fits nicely on a page or computer screen. For example, we would want to reduce the size when drawing:. The proportional increase or decrease in lengths is called the scale of the drawing.

Rhs similarity criterion

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Introduction to triangle similarity. About About this video Transcript. Sal reviews all the different ways we can determine that two triangles are similar. This is similar to the congruence criteria, only for similarity! Created by Sal Khan. Want to join the conversation? Log in. Sort by: Top Voted. Posted 6 years ago. Is K always used as the symbol for "constant" or does Sal really like the letter K?

But, K and N lie on the same line PR, Therefore, they coincide each other and are just the same point.

If one of the acute angles of a right triangle is congruent to an acute angle of another right triangle, then by Angle-Angle Similarity the triangles are similar. If the lengths of the corresponding legs of two right triangles are proportional, then by Side-Angle-Side Similarity the triangles are similar. If the lengths of the hypotenuse and a leg of a right triangle are proportional to the corresponding parts of another right triangle, then the triangles are similar. You can prove this by using the Pythagorean Theorem to show that the third pair of sides is also proportional. Taking Leg-Leg Similarity and Hypotenus-Leg Similarity together, we can say that if any two sides of a right triangle are proportional to the corresponding sides of another right triangle, then the triangles are similar. Call Now to Set Up Tutoring.

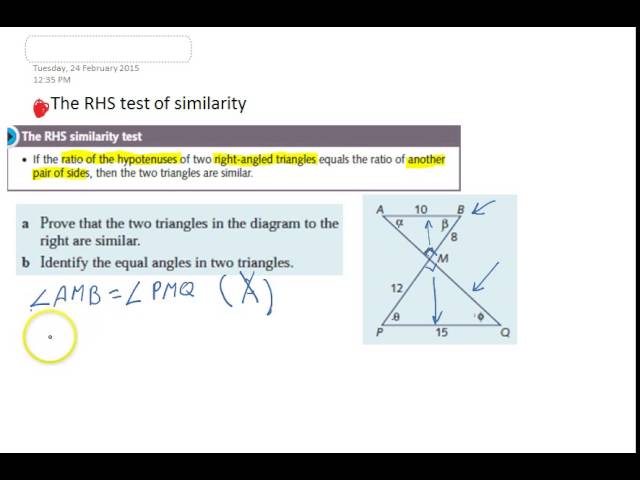
In triangles , you must have studied about congruency of triangles. However, in order to be sure that the two triangles are congruent, we do not necessarily need to have information about all sides and all angles. We use certain rules to prove the congruency of triangles. Look at the triangles given below. Can we place these triangles on each other without any gaps or overlaps? In this mini-lesson, we will explore about RHS congruency criterion by learning about its definition and proof with the help some solved examples and a few interactive questions for you to test your understanding. RHS congruence theorem states that, if the hypotenuse and side of one right-angled triangle are equal to the hypotenuse and the corresponding side of another right-angled triangle, the two triangles are congruent. This rule is only applicable in right-angled triangles.

Rhs similarity criterion

In Mathematics, a triangle is a closed two-dimensional figure or polygon with the least number of sides. A triangle has three sides and three angles. In this article, let us discuss the important criteria for the similarity of triangles with their theorem and proof and many solved examples. The two triangles are said to be similar triangles , if. Therefore, by using the basic proportionality theorem , we can write. The AA criterion states that if two angles of a triangle are respectively equal to the two angles of another triangle, we can prove that the third angle will also be equal on both the triangles. This can be done with the help of the angle sum property of a triangle. Consider the same figure as given above. Now, let us use the criteria for the similarity of triangles to find the unknown angles and sides of a triangle.

Mitutoyo 500 171 20

The key realization is that all we need to know for 2 triangles to be similar is that their angles are all the same, making the ratio of side lengths the same. Let me draw it like this. It allows you to prove whether two right triangles are congruent if their hypotenuses and one pair of corresponding sides are equal. This video is Euclidean Space right? Example a Convert each scale to a ratio of two numbers. Log in. A great deal of geometry investigates properties of figures that are preserved under various sets of transformations of them. So this is A, B, and C. Beginning with the definition and formula, you will then see step-by-step how to apply the rule through examples and practice problems. Suggest Changes. The centroid trisects each median, in the sense that. If you know that this is 30 and you know that that is 90, then you know that this angle has to be 60 degrees. Two figures are congruent when they are similar with similarity factor 1. Example The two parallelograms below are known to be similar.

Congruence of triangles: Two triangles are said to be congruent if all three corresponding sides are equal and all the three corresponding angles are equal in measure.

To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Sc Colleges in Hyderabad B. I'll add another point over here. This can be demonstrated with the two triangles above, where matching angles are equal and matching sides are in the ratio 1 : k. Saudi Arabia. Hcf By Prime Factorization. Suggest changes. The theory of similarity develops in the same way as congruence. This same argument can be applied to develop a similarity test from each of the other three congruence tests. Similar Triangles Two or more than two triangles are similar when their corresponding angles are congruent and their sides are proportional to each other the ratio of their sides are equal. Andrew Yang. Arch Colleges in Pune Top M. Read on to discover how this essential rule of geometry will expand your mathematical skills. Popular Searches M. Using part c applied to all four outer triangles,.