Length of chord formula class 10

Chord of a circle is a line segment that links two locations on the circumference of the circle. A circle is a two-dimensional shape where a set of all points are equally spaced from a fixed point in a plane. The fixed point is termed the center of the length of chord formula class 10. Diameter of the circle is a line that meets 2 points on the edge of the circle and goes through the center and distance surrounding the circle is termed circumference.

The chord of a circle can be stated as a line segment joining two points on the circumference of the circle. The diameter is the longest chord of the circle which passes through the center of the circle. The figure shown below represents the circle and its chord. In the circle above with center O, AB represents the diameter of the circle longest chord of a circle , OE represents the radius of a circle and CD represents the chord of a circle. Let us consider CD as the chord of a circle and points P and Q lying anywhere on the circumference of the circle. In this article, we will study what is a chord in a circle, chord length formulas, how to find the length of the chord, length of the common chord of two circles formulas, chord radius formulas, etc.

Length of chord formula class 10

Chord of a circle is the line that joints any two points on the circumference of the circle. A circle can have various chords and the largest chord of a circle is the diameter of the circle. We can easily calculate the length of the chord using the Chord Length Formula. As the name suggests it is the formula for calculating the length of the chord in a circle in Geometry. In this article, we will learn about the definition of the chord, theorems of the chords and the circle, explain its properties, and the formulas to calculate the length of the chord using different methods. The article also has some solved sample problems for better understanding. A circle is a perfect round shape consisting of all points in a plane that are placed at a given distance from a given point. They consist of a closed curved line around a central point. The points present on the line are at the same distance from the central point. The distance to the centre of a circle is called a radius. The line segment that joins any two points on the circumference of the circle is known as the chord of a circle. As the diameter also joins the two points on the circumference of a circle, thus it is also a chord to a circle.

In the above diagram, r is the radius of the circle, and d is the perpendicular distance from the chord to the center of the circle. Tangents will always be perpendicular to the radius of the given circle.

The chord of a circle is defined as the line segment joining any two points on the circumference of the circle. It should be noted that the diameter is the longest chord of a circle that passes through the center of the circle. A line segment that joins two points on the circumference of the circle is defined as the chord of the circle. Among the other line segments that can be drawn in a circle, the chord is one whose endpoints lie on the circumference. Observe the following circle to identify the chord PQ. Diameter is also considered to be a chord which passes through the center of the circle.

The chord of any circle is an important term. It is defined as the line segment joining any two points on the circumference of the circle, not passing through its centre. Therefore, the diameter is the longest chord of a given circle, as it passes through the centre of the circle. Calculation of the length of the chord is sometimes very important in mathematics. This article will explain the chord length formula with examples. Let us learn it! A chord is the line segment in a circle, which connects any two points on the circumference of the circle. The same two points are connected by the curve in the form of the corresponding arc in the circle. We may also calculate the chord length if we know both the radius and the length of the right bisector.

Length of chord formula class 10

The radius of is feet and. Find the length of chord. We begin by drawing in three radii: one to , one to , and one perpendicular to with endpoint on our circle. We must also recall that our central angle has a measure equal to its intercepted arc. Our perpendicular radius actually divides into two congruent triangles.

Cumflation comics

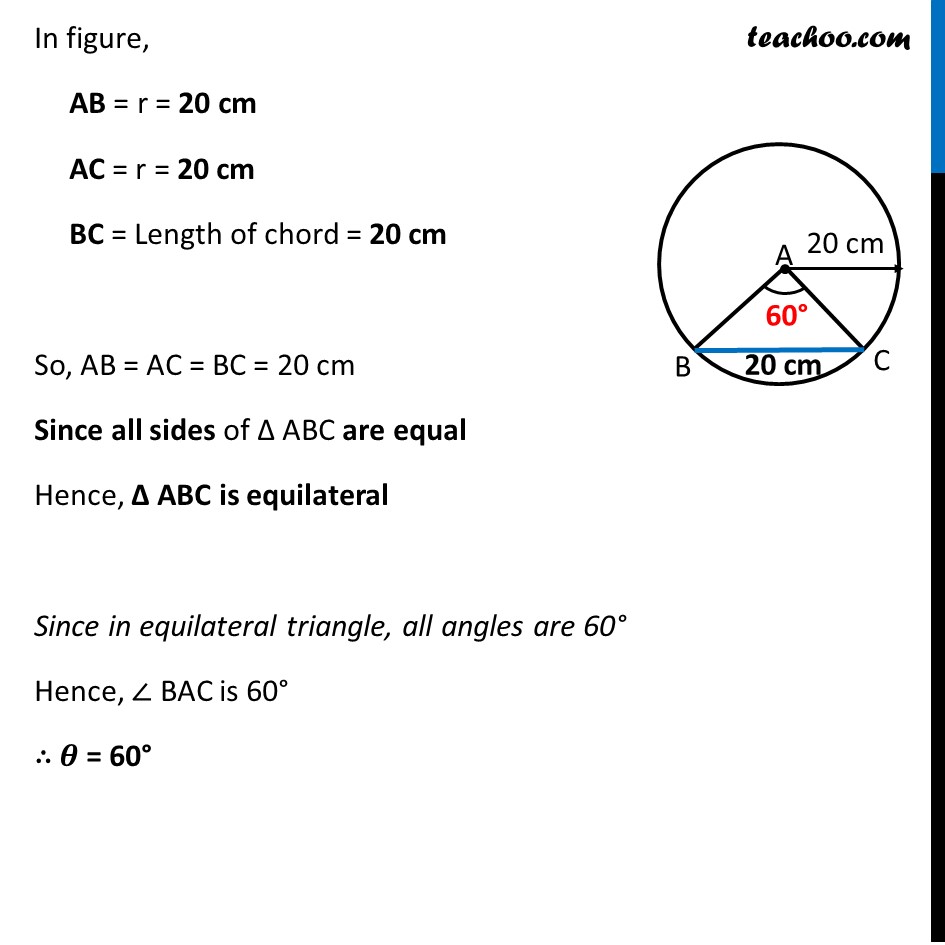
You will be notified via email once the article is available for improvement. Previous Sequences and Series Formulas. Easy Normal Medium Hard Expert. Hi there! Two chords are identical in length if they are at an equal distance from the center of a circle. Find the angle subtended by this chord at a point in the major segment. Problem 3: A circle is an angle of 60 degrees whose radius is 12cm. Last updated on May 3, The chord radius formula when length and height of the chord are given is. The diameter is the longest chord of the circle which passes through the center of the circle.

The chord of a circle can be defined as the line segment joining any two points on the circumference of the circle.

Previous Sequences and Series Formulas. Thus, the chord length for any circle with its perpendicular distance from the centre is known is given as. Chord of Circle The chord of a circle is defined as the line segment joining any two points on the circumference of the circle. However, the chords that are not at equal distance from the center of a circle are supposed to be unequal chords. It is equal to twice the radius of the circle. Diameter of a Circle: The diameter of a circle is a straight line passing through the center of the circle that connects the 2 points on the boundary. Last Updated : 24 Jan, Change Language. A chord is a line segment that joins any two points on the circumference of the circle. This is also recognized as the equal angles equal chords theorem or converse of theorem 1. Engineering Exam Experiences. Join courses with the best schedule and enjoy fun and interactive classes. Become a problem-solving champ using logic, not rules. The longest chord of a circle is its diameter. Chords of a Circle.