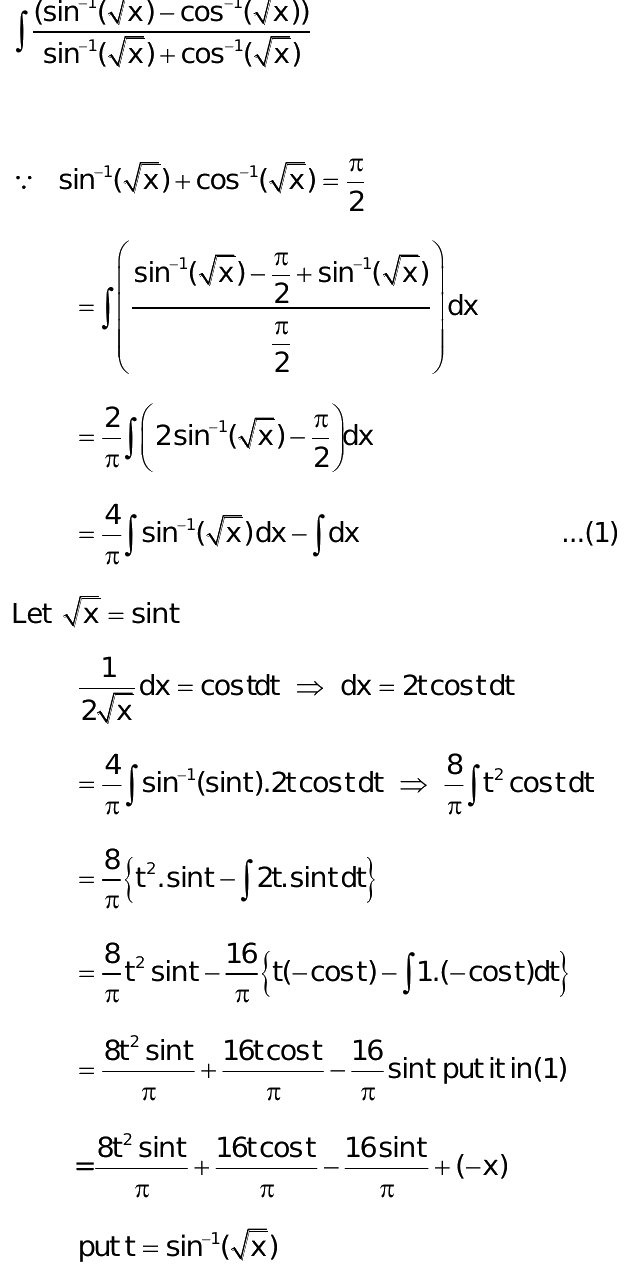
Integration of cos root x

We have already encountered and evaluated integrals containing some expressions of this type, but many still remain inaccessible.

The trigonometric substitutions we will focus on in this section are summarized in the table below:. Functions consisting of powers of the sine and cosine can be integrated by using substitution and trigonometric identities. These can sometimes be tedious, but the technique is straightforward. A similar technique is applicable to powers of secant and tangent as shown in Section 2. An example will suffice to explain the approach.

Integration of cos root x

Before going to find the integral of cos x, let us recall what is integral. An integral is nothing but the anti-derivative. Anti-derivative, as its name suggests, can be found by using the reverse process of differentiation. Thus, the integration of cos x is found by using differentiation. Let us see more about the integral of cos x along with its formula and proof in different methods. The integral of cos x dx is sin x. But how to do the integration of cos x? We are going to find the integral of cos x in various methods such as using the derivatives and using the substitution method in the upcoming sections. The integration is the opposite reverse process of differentiation. So to find the integral of cos x, we have to see by differentiating what function would give us cos x. Let us recall the formulas of differentiation and search for some formula that gives us cos x as the derivative. Thus, the derivative of sin x is cos x. So the integration of cos x anti-derivative must be sin x.

As an Amazon Associate we earn from qualifying purchases. An example will suffice to explain the approach.

In mathematics , a nonelementary antiderivative of a given elementary function is an antiderivative or indefinite integral that is, itself, not an elementary function i. Some common non-elementary antiderivative functions are given names, defining so-called special functions , and formulas involving these new functions can express a larger class of non-elementary antiderivatives. The examples above name the corresponding special functions in parentheses. Nonelementary antiderivatives can often be evaluated using Taylor series. Even if a function has no elementary antiderivative, its Taylor series can always be integrated term-by-term like a polynomial , giving the antiderivative function as a Taylor series with the same radius of convergence. However, even if the integrand has a convergent Taylor series, its sequence of coefficients often has no elementary formula and must be evaluated term by term, with the same limitation for the integral Taylor series. Even if it is not possible to evaluate an indefinite integral antiderivative in elementary terms, one can always approximate a corresponding definite integral by numerical integration.

In this section we look at how to integrate a variety of products of trigonometric functions. These integrals are called trigonometric integrals. They are an important part of the integration technique called trigonometric substitution , which is featured in Trigonometric Substitution. This technique allows us to convert algebraic expressions that we may not be able to integrate into expressions involving trigonometric functions, which we may be able to integrate using the techniques described in this section. In addition, these types of integrals appear frequently when we study polar, cylindrical, and spherical coordinate systems later. For integrals of this type, the identities. After applying these formulas, simplify and reapply strategies 1 through 3 as appropriate. Use strategy 2. These formulas may be derived from the sum-of-angle formulas for sine and cosine. As we see in the following example, we can evaluate these new integrals by using u-substitution.

Integration of cos root x

Wolfram Alpha is a great tool for calculating antiderivatives and definite integrals, double and triple integrals, and improper integrals. The Wolfram Alpha Integral Calculator also shows plots, alternate forms and other relevant information to enhance your mathematical intuition. Use Math Input above or enter your integral calculator queries using plain English. To avoid ambiguous queries, make sure to use parentheses where necessary. Here are some examples illustrating how to ask for an integral using plain English. Get immediate feedback and guidance with step-by-step solutions for integrals and Wolfram Problem Generator. The indefinite integral of , denoted , is defined to be the antiderivative of. In other words, the derivative of is. Since the derivative of a constant is 0, indefinite integrals are defined only up to an arbitrary constant.

Steinbachonline com

To calculate the approximate areas, we drew the triangles. In this case, the integral becomes. Evaluate the following indefinite integrals. Kindergarten Worksheets. Graphical Intuition of Integral of Cos x. Table of contents. If so, we may wish to consider applying an alternative technique. The examples above name the corresponding special functions in parentheses. Show that the results are equivalent. Find the volume of the solid that is generated. We have to add an integration constant after integrating any function. Thus, the derivative of sin x is cos x.

Integral of cos sqrt x along with its formula and proof with examples. In calculus, the integral calculator follows a fundamental concept that assigns numbers to functions to define displacement, area, volume, and all those functions that contain a combination of tiny elements.

This technique works for products of powers of sine and cosine. Anti-derivative, as its name suggests, can be found by using the reverse process of differentiation. Our Mission. The integration is the opposite reverse process of differentiation. So the integration of cos x anti-derivative must be sin x. An example will suffice to explain the approach. Solution The Guideline doesn't help us in this scenario. If so, we may wish to consider applying an alternative technique. Are the results the same? Learn Practice Download. Maths Games. Maths Program.