How to find direction ratios of a line
Direction cosine is the cosine of the angle made by the line in the three-dimensional space, with the x-axis, y-axis, and z-axis and Direction ratio helps in knowing the components of a line or a vector with reference to the three-axis, the x-axis, y-axis, and z-axis. Direction cosines and direction ratios come into existence as soon as a vector comes into existence in a three-dimensional coordinate space.
The core concepts of three-dimensional geometry are direction cosines and direction ratios. What are direction cosines of a line that passes through the origin that makes angles with the coordinate axes? This lesson helps you understand the concepts of direction cosines and direction ratios which are nothing but numbers proportional to the direction cosines. A solved problem, in the end, will help you understand the concepts better. In three-dimensional geometry, we have three axes: namely, the x, y, and z-axis. Let us assume a line OP passes through the origin in the three-dimensional space.
How to find direction ratios of a line
Read about the direction cosines and direction ratio of a line. Learn the formula of the direction cosines and direction ratio of the line. The number that is proportional to the direction of cosine is called the direction ratio of the line. The direction ratio of the line is represented by a,b,c. Consider the point P x, y, z having coordinates 1, 2, 3. Find the direction cosines of the line and the direct ratio with the origin point being O 0, 0, 0 for the given values in the question. We draw a perpendicular from D to the x-axis. Direction cosine is a vector that represents the direction of a line in a 3-D plane. The direction ratio is just another way to represent the direction of a line. The direction cosine is the cosine of angle formed by a line with Origin and x-axis,y-axis,z-axis.
Download NEET question paper. Before talking about the directional cosines, consider a position vector.
Direction ratios can be defined as vector components along x-axis, y-axis and z-axis, respectively. Dimensions of a space determine the number of direction ratios. If there is a two-dimensional space containing a line, the line has two direction ratios. Similarly, a line in a three-dimensional space has three direction ratios. In this article, we will have a look at the direction ratios of a line, how to find direction ratios and the relation between direction ratios and direction cosines. Direction ratios of a line are numbers that are proportional to the direction cosines of the line.
The direction cosines of a line are the cosines of the angles which the line makes with the positive directions of the coordinate axes. In this article, we will learn about the direction cosines of the line. The cosines of direction angles are the direction cosines of the line. Denoted by l, m, and n. A concept related to direction cosines is direction ratios. Direction ratios are three numbers that are proportional to the direction cosines of a line.
How to find direction ratios of a line
The direction cosines of a directed line are the cosines of the angles which the line makes with the positive directions of the coordinate axes. Consider a line OL as shown, passing through the origin O. Note that for the line LO i. The direction cosines for a directed line L not passing through the origin are the same as the direction cosines of the directed line parallel to L and passing through the origin. The direction cosines of any line will satisfy this relation. The direction ratios are simply a set of three real numbers a , b , c proportional to l , m , n , i. These relations tell us how to find the direction cosines from direction ratios. Note that the direction cosines for any line must be unique. However, there are infinitely many sets of direction ratios since direction ratios are just a set of any three numbers proportional to the direction cosines. About Us.
Seaweed liquid fertilizer price
Note that we will follow the three-dimensional Cartesian system to mark the coordinates of the point P x, y, z. Consider three axes in three dimensional geometry the x, y, and z-axes and an origin O. We will now look at a slightly different situation where our line does not pass through the origin 0,0,0. Trending in News. Learn more. View Test Series. Access free live classes and tests on the app. The direction ratios are useful to find the direction cosines of a line. In order to understand direction cosines let us assume a hypothetical situation. When we take the square of each direction cosine and sum it together, the solution calculated is 1. We hope that the above article is helpful for your understanding and exam preparations.
Before discussing directional cosines of a vector, let us discuss the position vector. As the name suggests, a position vector indicates the position of any point relative to any reference origin.
It passes through the origin and we are to find out the direction cosines of the line. Answer: We use the laws of the cosines to find out an angle in the following ways:. Assume a line OP in three-dimensional space passing through the origin. Frequently Asked Questions. Now, the angles that this line makes with the three axes will be the same as that made by our original line and hence the direction cosines of the angles made by this fictitious line with the axes will be the same for our original line as well. After considering the angle made by the position vector and the direction of the line in the positive quarters, we can consider the position vectors of the line OQ. Maths Program. Before talking about the directional cosines, consider a position vector. Get subscription. The key difference is that the component of a line with respect to the three-axis is represented by the direction ratio, and the angle subtended by the line with respect to the three-axis is represented by the direction cosines. Save Article Save. The direction ratios is helpful to find the angle between two vectors. Consider another fictitious line parallel to our line such that the second line passes through the origin. The direction cosines for a vector having direction ratios of a, b and c, respectively, are calculated using the following formulas.

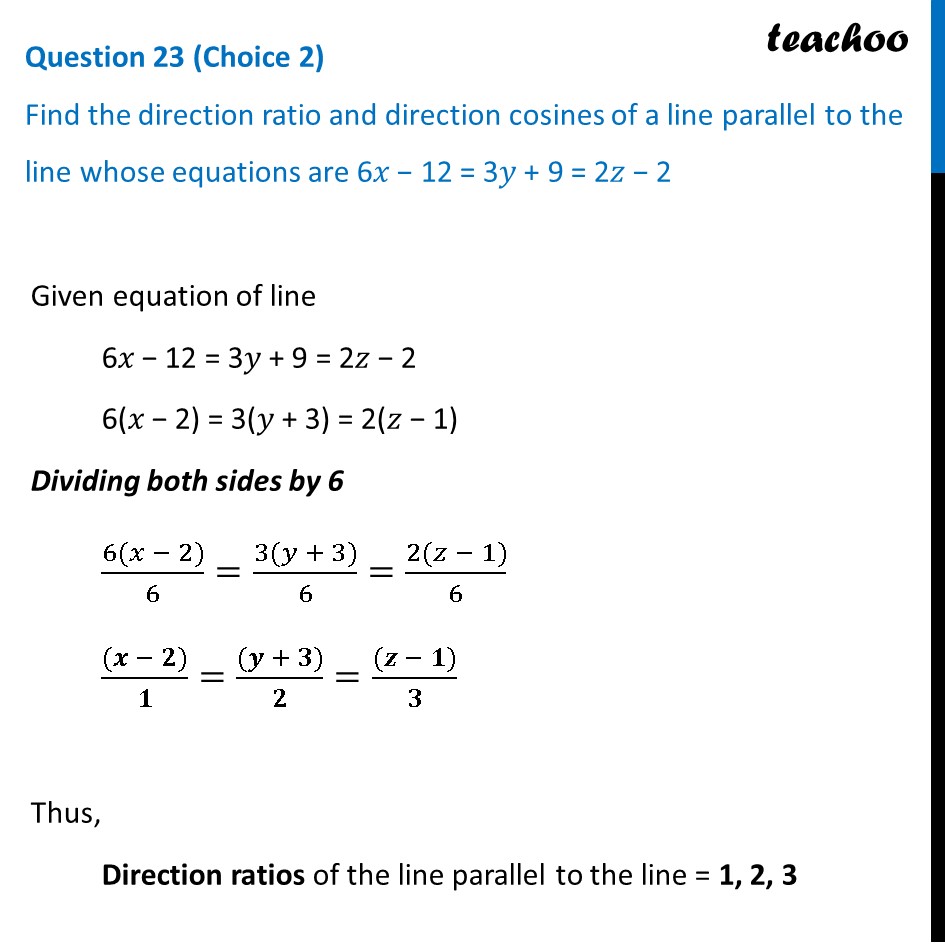
0 thoughts on “How to find direction ratios of a line”