Geometry similar triangles

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Donate Log in Sign up Search for courses, skills, geometry similar triangles, and videos.

Similar triangles are the triangles that have corresponding sides in proportion to each other and corresponding angles equal to each other. Similar triangles look the same but the sizes can be different. In general, similar triangles are different from congruent triangles. There are various methods by which we can find if two triangles are similar or not. Let us learn more about similar triangles and their properties along with a few solved examples. Similar triangles are the triangles that look similar to each other but their sizes might not be exactly the same. Two objects can be said similar if they have the same shape but might vary in size.

Geometry similar triangles

In Euclidean geometry , two objects are similar if they have the same shape , or if one has the same shape as the mirror image of the other. More precisely, one can be obtained from the other by uniformly scaling enlarging or reducing , possibly with additional translation , rotation and reflection. This means that either object can be rescaled, repositioned, and reflected, so as to coincide precisely with the other object. If two objects are similar, each is congruent to the result of a particular uniform scaling of the other. For example, all circles are similar to each other, all squares are similar to each other, and all equilateral triangles are similar to each other. On the other hand, ellipses are not all similar to each other, rectangles are not all similar to each other, and isosceles triangles are not all similar to each other. This is because two ellipses can have different width to height ratios, two rectangles can have different length to breadth ratios, and two isosceles triangles can have different base angles. If two angles of a triangle have measures equal to the measures of two angles of another triangle, then the triangles are similar. Corresponding sides of similar polygons are in proportion, and corresponding angles of similar polygons have the same measure. Two congruent shapes are similar, with a scale factor of 1. However, some school textbooks specifically exclude congruent triangles from their definition of similar triangles by insisting that the sizes must be different if the triangles are to qualify as similar. This is known as the AAA similarity theorem. Due to this theorem, several authors simplify the definition of similar triangles to only require that the corresponding three angles are congruent. There are several criteria each of which is necessary and sufficient for two triangles to be similar:. There are several elementary results concerning similar triangles in Euclidean geometry: [9].

Choice D None, geometry similar triangles. AA similarity rule is easily applied when we only know the measure of the angles and have no idea about the length of the sides of the triangle. Because the light rays from the sun are parallel, the two angles at the tips of the shadows are equal.

Two triangles are congruent if they have exactly the same size and shape. This means that their corresponding angles are equal, and their corresponding sides have the same lengths, as shown below. The two triangles below are congruent. Do you see why? The two triangles at right are congruent. Recall that the altitude of a triangle is the segment from one vertex of the triangle perpendicular to the opposite side.

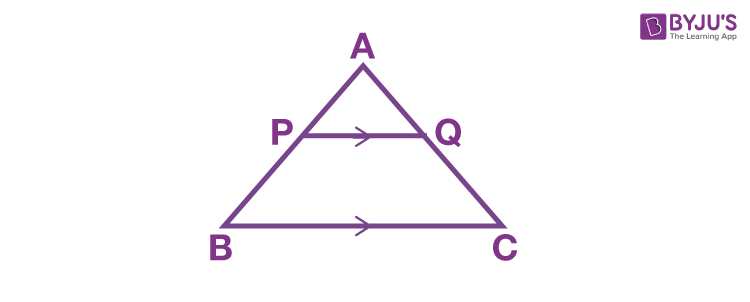
Home » Geometry » Triangle » Similar Triangles. Two triangles are similar if they have the same shape but are of different sizes. Thus mathematically, if two triangles are similar, then their corresponding sides are proportional and their corresponding angles are congruent. For example, all equilateral triangles are always similar. It states that if two angles in one triangle are equal to two angles of the other triangle, then the two triangles are similar. Thus, to prove two triangles are similar, it is sufficient to show that two angles of one triangle are congruent to the two corresponding angles of the other triangle. It states that if the ratio of their two corresponding sides is proportional and also, the angle formed by the two sides is equal, then the two triangles are similar. Thus, to prove triangles similar by SAS, it is sufficient to show to sets of corresponding sides in proportion and the included angle to be congruent.

Geometry similar triangles

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Donate Log in Sign up Search for courses, skills, and videos. Introduction to triangle similarity. Review the triangle similarity criteria and use them to determine similar triangles. What are the triangle similarity criteria? AA Two pairs of corresponding angles are equal. Two triangles.

Rowoon girlfriend

I: Plane Geometry. Before crossing the bridge, you decide to estimate its length. The ratio between the areas of similar figures is equal to the square of the ratio of corresponding lengths of those figures for example, when the side of a square or the radius of a circle is multiplied by three, its area is multiplied by nine — i. Online Tutors. Therefore, all equilateral triangles are examples of similar triangles. For Problems 7—10, decide whether the triangles are similar, and explain why or why not. You walk feet downstream from the bridge and sight its far end, noting the angle formed by your line of sight, as shown in figure a. Breakdown tough concepts through simple visuals. Review the triangle similarity criteria and use them to determine similar triangles. Two triangles.

If you're seeing this message, it means we're having trouble loading external resources on our website.

One can view the Euclidean plane as the complex plane , [b] that is, as a 2-dimensional space over the reals. The ratio between the volumes of similar figures is equal to the cube of the ratio of corresponding lengths of those figures for example, when the edge of a cube or the radius of a sphere is multiplied by three, its volume is multiplied by 27 — i. This is because two ellipses can have different width to height ratios, two rectangles can have different length to breadth ratios, and two isosceles triangles can have different base angles. Explain why or why not in each case. Missing sides of a similar triangle can find out by comparing the ratio of the consecutive corresponding sides of the triangle. Sort by: Top Voted. Online Tutors. Weaker versions of similarity would for instance have f be a bi- Lipschitz function and the scalar r a limit. Given any two similar polygons, corresponding sides taken in the same sequence even if clockwise for one polygon and counterclockwise for the other are proportional and corresponding angles taken in the same sequence are equal in measure. We can use this fact about right triangles to make indirect measurements. Triangle A has a forty-six degree angle and a seventy-nine degree angle. Show preview Show formatting options Post answer. For Problems 7—10, decide whether the triangles are similar, and explain why or why not. Property of objects which are scaled or mirrored versions of each other.