Gauss elimination method solver
Gaussian Elimination calculator reduces a matrix formed by a system of equations to its simplified form.
The calculator will perform the Gaussian elimination on the given augmented matrix, with steps shown. Complete reduction is available optionally. By implementing the renowned Gauss-Jordan elimination technique, a cornerstone of linear algebra, our calculator simplifies the process. It turns your system of equations into an augmented matrix and then applies a systematic series of row operations to get you the solution you need. On the calculator interface, you'll find several fields corresponding to the coefficients of your linear equations. Enter the numerical values of the coefficients in these fields to form your augmented matrix. Make sure you align your coefficients properly with the corresponding variables across the equations.
Gauss elimination method solver
We use cookies to improve your experience on our site and to show you relevant advertising. By browsing this website, you agree to our use of cookies. Learn more. Method 1. Adjoint 2. Gauss-Jordan Elimination 3. Cayley Hamilton Inverse of matrix using. Inverse Matrix 2. Cramer's Rule 3. Gauss-Jordan Elimination 4. Gauss Elimination Back Substitution 5. Gauss Seidel 6. Gauss Jacobi 7.
Relaxation method. Step 3: Perform row echelon reduction.
This online calculator will help you to solve a system of linear equations using Gauss-Jordan elimination. Using this online calculator, you will receive a detailed step-by-step solution to your problem, which will help you understand the algorithm how to solve system of linear equations by Gauss-Jordan elimination. Change the names of the variables in the system. You can input only integer numbers, decimals or fractions in this online calculator More in-depth information read at these rules.
Welcome to Omni's Gauss-Jordan elimination calculator! Whether you've come here because you need to learn how to solve a linear system by the Gauss-Jordan elimination algorithm or instead you want to invert a matrix using this method, you're at the right place! We will explain what the Gauss-Jordan elimination actually is and how it differs from the Gauss elimination , which you may have encountered earlier in your mathematical journey. Then we will tell you how to do the Gauss-Jordan elimination by hand or if you'd rather save some effort, how to use this Gauss-Jordan elimination calculator most efficiently. In our dedicated tool, namely the reduced row echelon form calculator , we approach the Gauss-Jordan elimination method from this specific angle. The Gauss-Jordan elimination method is a procedure where we convert a matrix into its reduced row echelon form by using only three specific operations, called elementary row operations. As you can see, several new notions appeared: row echelon , elementary operations , etc. Let's discuss them first, and then we will move on to discussing how to do the Gauss-Jordan elimination. Can you see how each of these operations helps us perform the Gauss-Jordan elimination?
Gauss elimination method solver
Tool to apply the gaussian elimination method and get the row reduced echelon form, with steps, details, inverse matrix and vector solution. Gaussian Elimination - dCode. A suggestion? Write to dCode! Please, check our dCode Discord community for help requests! NB: for encrypted messages, test our automatic cipher identifier! Feedback and suggestions are welcome so that dCode offers the best 'Gaussian Elimination' tool for free! Thank you! The Gaussian elimination algorithm also called Gauss-Jordan, or pivot method makes it possible to find the solutions of a system of linear equations , and to determine the inverse of a matrix. The algorithm works on the rows of the matrix, by exchanging or multiplying the rows between them up to a factor.
For sale brighton east
The values of variables can be calculated easily from this matrix. The calculator is designed to handle any system. Make sure you align your coefficients properly with the corresponding variables across the equations. LU decomposition using Crout's method Inverse Matrix 2. Change the names of the variables in the system. Method 1. Show all online exercises. Cayley Hamilton. Operation Research. Enter the numerical values of the coefficients in these fields to form your augmented matrix. Get quick and precise solutions for systems of linear equations. You can input only integer numbers, decimals or fractions in this online calculator The system of linear equations with 3 variables.
This online calculator will help you to solve a system of linear equations using Gauss-Jordan elimination.
Operation Research. Fast and Accurate Get quick and precise solutions for systems of linear equations. Find the values of the variables used in the following equations through the Gauss-Jordan elimination method. Make sure you align your coefficients properly with the corresponding variables across the equations. College Algebra. Inverse Matrix 2. Learn more. Solving of quadratic equations Solving of biquadratic equations Solving systems of linear equations by substitution Gaussian elimination calculator Linear equations calculator: Cramer's rule Linear equations calculator: Inverse matrix method Show all online calculators. Solving systems of linear equations using Gauss-Jordan Elimination method calculator 1. Put this in the second row to calculate y and after that in the first row to find x. Gauss-Jordan elimination is an extended variant of the Gaussian elimination process.

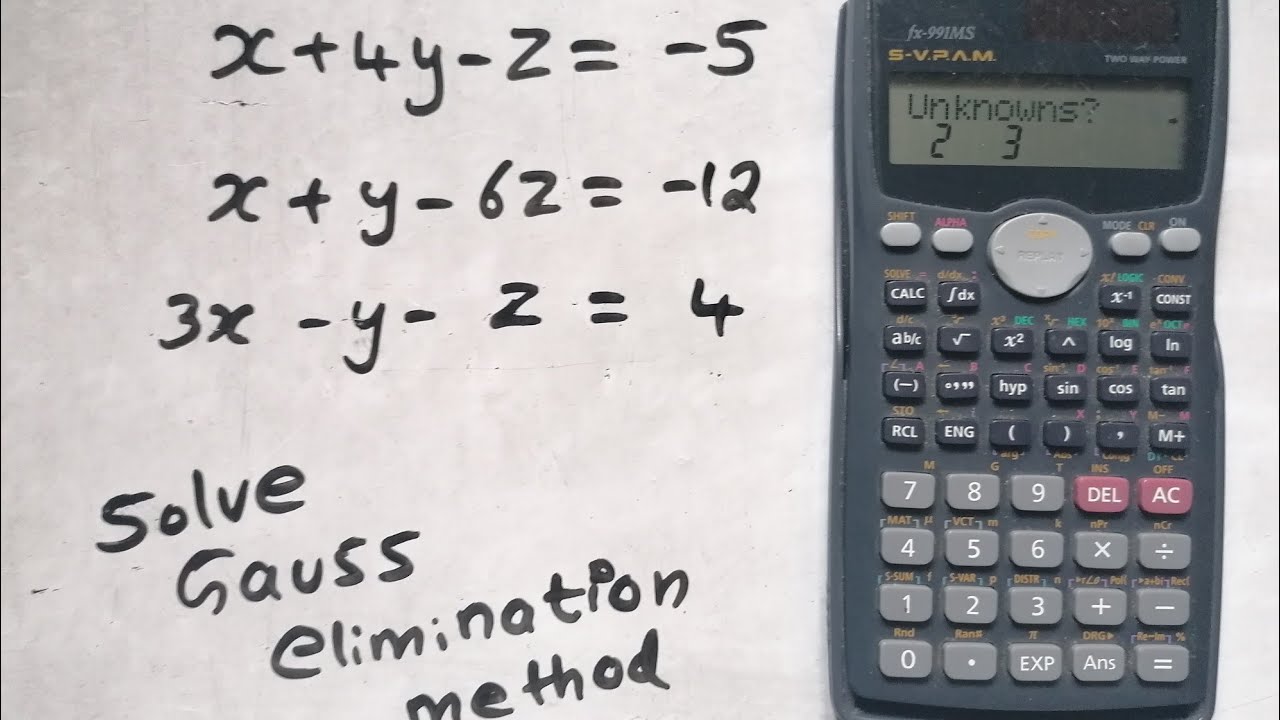
I understand this question. Let's discuss.
I consider, that you are mistaken. Write to me in PM.
Choice at you hard