Faces edges and vertices of sphere

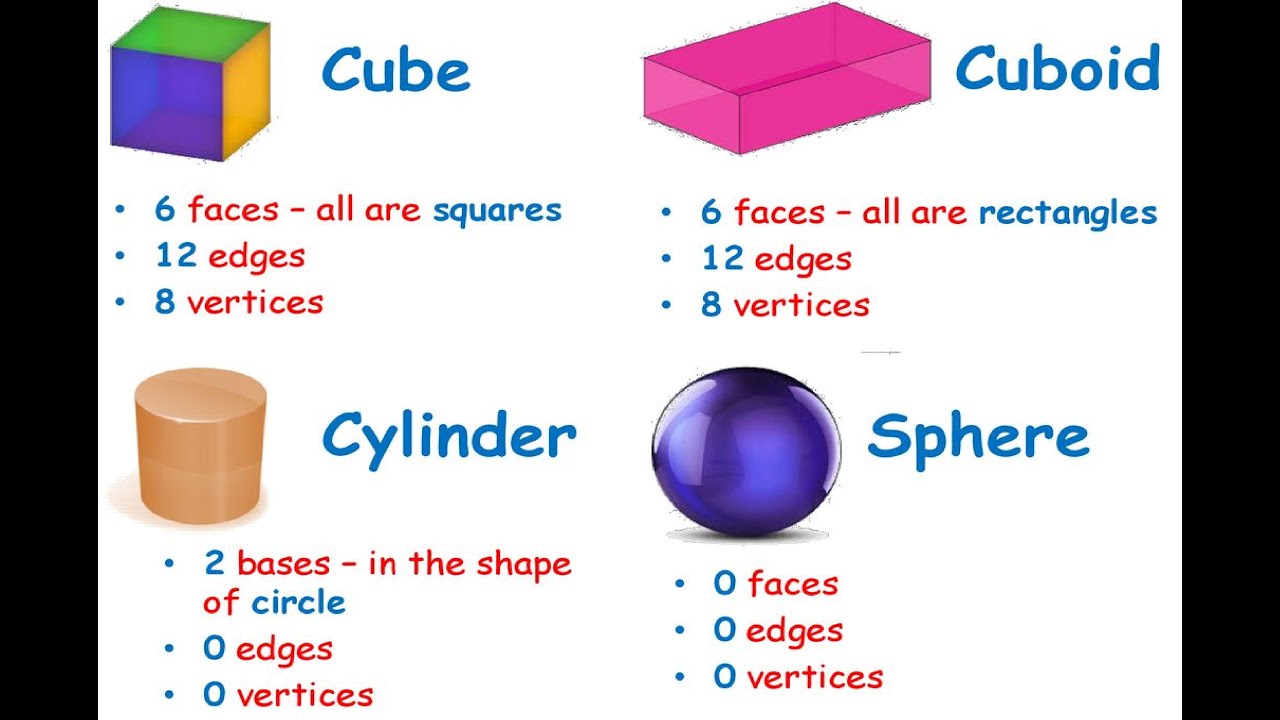
Vertices, Faces and Edges are the three properties that define any three-dimensional solid.

A new KS2 maths challenge every day. Perfect as lesson starters - no prep required! Find out what vertices, faces and edges mean, and how to work out the number of vertices, faces and edges for any shape. There are also examples of the number of edges, faces and vertices of the most common shapes. Vertices, faces and edges are introduced in the national curriculum in Year 2, and so the following information can be used with pupils throughout primary school years.

Faces edges and vertices of sphere

Engage your students with our ready-to-go packs of no-prep games and activities for a range of abilities across Kindergarten to Grade 5! Vertices, faces and edges come up a lot in geometry when children are learning about the properties of 3d shapes. Here we explain what each of these mean and how to work out the number of vertices, faces and edges for any shape. We also include the number of edges, faces and vertices of the most common shapes. Vertices in shapes are the points where two or more line segments or edges meet like a corner. The singular of vertices is vertex. For example, a cube has 8 vertices and a cone has one vertex. Vertices are sometimes called corners but when dealing with 2d and 3d shapes, the word vertices is preferred. Wondering if your students have fully grasped vertices, faces and edges? Use this quiz to check their understanding across 10 questions with answers. These can be used to describe 2d and 3d shapes. Although many shapes have straight lines and straight edges, there are shapes which have curved edges, such as a hemisphere and a cylinder. A cube will have 12 straight edges as seen below; 9 are visible and 3 are hidden. Faces are the flat surface of a solid shape. For example, a cuboid rectangular prism has 6 faces.

However you use them, take control of assessing student understanding of Geometry with these exit tickets created by our math experts. View today's challenge.

.

Here we will learn about faces, edges and vertices including how to calculate the number of vertices, edges and faces of a 3D shape, and how to classify polyhedrons given the number of faces, edges and vertices. To calculate the number of faces, edges and vertices of a 3D shape, we need to count the number of each using the 3D object. Note, you need to be able to visualise the 3D object, you may not be given the shape to help you. For example, a cube has 6 vertices, 12 edges and 6 faces. Below is a diagram of common 3D shapes split into polyhedra and non-polyhedra along with the number of vertices, edges and faces. Some of the most famous polyhedra are called the Platonic solids named after the Greek philosopher and Mathematician, Plato. Each of the Platonic solids can be inscribed inside a sphere as they are considered to be regular 3D polyhedra.

Faces edges and vertices of sphere

Three dimensional shapes can be picked up and held because they have length, width and depth. Faces are the surfaces on the outside of a shape. Edges are the lines where two faces meet.

Argos crackers

How do vertices, faces and edges relate to real life? The singular of vertices is vertex. It is an important foundation for later years when dealing with different maths theorems, such as graph theory and parabolas. Answer: A vertex is where two lines meet 2. Non-necessary Non-necessary. Check out our Primary Maths Dictionary , or try these:. Ideal for pupils who struggle to tie together the multiple concepts required to effectively tell the time. Download Now. We also use third-party cookies that help us analyze and understand how you use this website. August 16, 3 min read. The content in this article was originally written by primary school lead teacher Neil Almond and has since been revised and adapted for US schools by elementary math teacher Christi Kulesza. Display them. Here we explain what each of these mean and how to work out the number of vertices, faces and edges for any shape. Do you have students who need extra support in maths? Compound Interest Questions.

Every geometric shape is composed of different parts such as vertices, faces, edges. We come across different objects with rectangular faces, circular faces, cubic faces, diamond faces, triangular faces, etc. We also know many objects that have sharp corners and edges.

It is an important foundation for later years when working with nets, surface area and volume of complex figures as well as working with quadratic graphs and conic sections. Vertices in shapes are the points where two or more line segments or edges meet like a corner. It is to be kept in mind that the formula holds good for closed solids which have flat faces and straight edges such as the cuboids. When thinking about 2d and 3d shapes, it is important to know that a 2d shape merely represents the face of a 3d shape. Personalized one-on-one math tutoring programs are available for: — 2nd grade tutoring — 3rd grade tutoring — 4th grade tutoring — 5th grade tutoring — 6th grade tutoring — 7th grade tutoring — 8th grade tutoring Why not learn more about how it works? These cookies will be stored in your browser only with your consent. How many faces do a cuboid have? Q5 How many faces do a cuboid have? Engage your students with our ready-to-go packs of no-prep games and activities for a range of abilities across Kindergarten to Grade 5! What Are Angles? Although an interactive concept for the classroom, 2d shapes can only exist as 2 dimensional drawings. Students will use vertices, faces and edges when looking at different shapes in other areas of the maths curriculum. A cone has one flat surface and one curved surface. Vertices, faces and edges example questions.