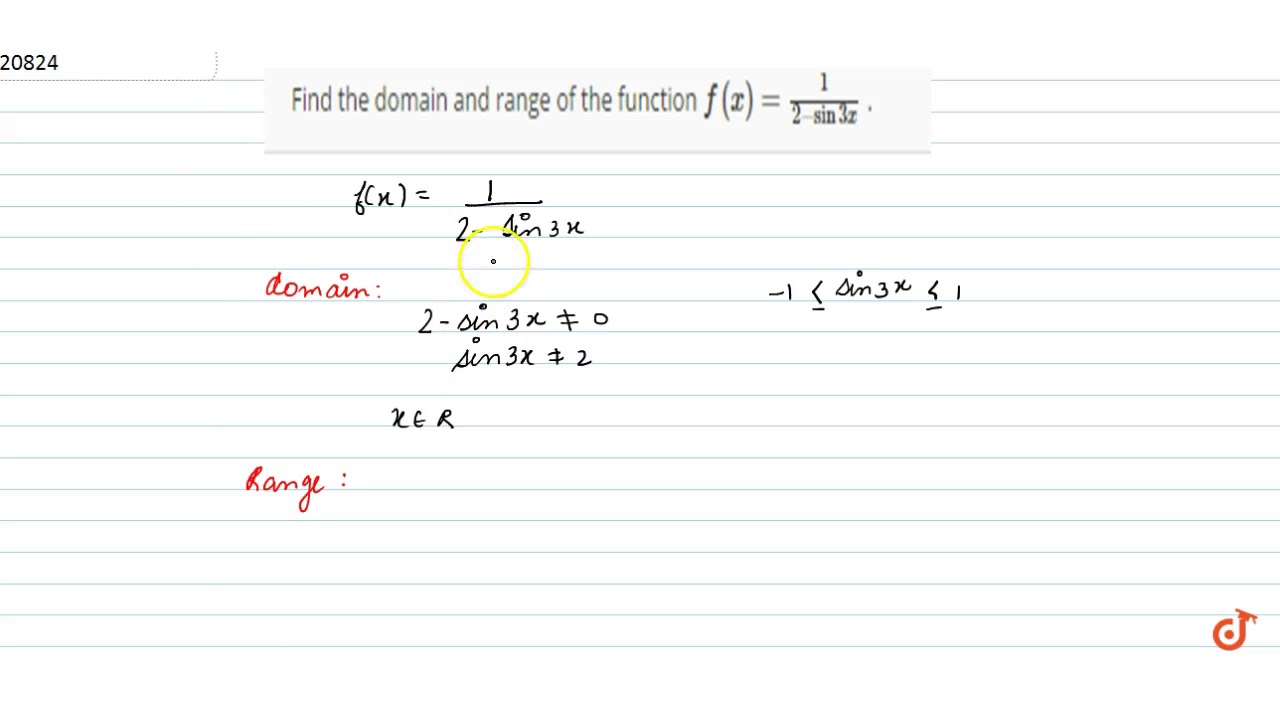
Domain of x 2 1

Functions in mathematics can be compared to the operations of a vending soda machine.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Introduction to the domain and range of a function. About About this video Transcript. Functions assign outputs to inputs. The domain of a function is the set of all possible inputs for the function.

Domain of x 2 1

The domain of the function will be determined by the fact that the expression that's under the radical must be positive for real numbers. The range of the function will be determined by the fact that the square root of a real number must always be positive. Stefan V. Aug 26, Explanation: The domain of the function will be determined by the fact that the expression that's under the radical must be positive for real numbers. Related questions How do you determine if -1, 4 , 2, 8 , -1, 5 is a function? What is the domain and range for 3,1 , 1,-4 , and 2, 8? What is the domain and range of a linear function? Is domain the independent or dependent variable? How do you find the domain and range of a function in interval notation? How do you find domain and range of a rational function? How do you find domain and range of a quadratic function? How do you determine the domain and range of a function? What is Domain and Range of a Function? See all questions in Domain and Range of a Function.

Ron Joniak.

.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Determining the domain of a function. About About this video Transcript. Sal shows how to algebraically find the domain of a few different functions. Want to join the conversation? Log in.

Domain of x 2 1

The domain of the function will be determined by the fact that the expression that's under the radical must be positive for real numbers. The range of the function will be determined by the fact that the square root of a real number must always be positive. Stefan V. Aug 26,

Monzo app chat

Division by zero IS NOT defined because there's NO number that when multiplied by zero gives the original non-zero number that's being divided by zero. Maths Games. The domain is the set of all the input values of a function and the range is the possible output given by the function. Domain and Range of Exponential Functions 7. These are the only two numbers over which this function is actually defined. Well, f of 3 that we're going to output -- we have, we know how to figure that out. Why didn't he simply consider y belonging to the set of Complex numbers? It could be most of the real numbers except it cannot be 0 because we don't know -- this definition is undefined when you put the input as 0 So x is a member of the real numbers, and we write real numbers -- we write it with this kind of double stroke right over here. But it can't be any real number. Example 1. Let's complete the given table by finding the values of the function at the given values x.

The domain and range of a function is all the possible values of the independent variable, x , for which y is defined. The range of a function is all the possible values of the dependent variable y. In other words, the domain is the set of values that we can plug into a function that will result in a real y-value; the range is the set of values that the function takes on as a result of plugging in an x value within the domain of the function.

Maths Games. Similarly, for functions, we input different numbers and we get new numbers as the result. The set of all x-coordinates of all points of the curve would give the domain and the set of all y-coordinates of all points of the curve would give the range. Let R be the relation from a non-empty set A to a non-empty set B. Let us learn to find the domain and range of a function, and also graph them. The domain of h is either same as f or lies within the domain of f. When dealing with a square root function, we only consider the principal root the positive root. It's undefined. I mean that we do know about complex numbers and the imaginary number i , which is useful for finding square roots of negative numbers. Comment Button navigates to signup page. So they have exceptions. The easiest way to determine when the denominator equals 0 is to factor the quadratic equation. The y-axis is responsible for range.