Critical points calc

Please ensure that your password is at least 8 characters and contains each of the following:. Enter a problem Hope that helps! You'll be able to enter critical points calc problems once our session is over.

The calculator will try to find the critical stationary points, the relative local and absolute global maxima and minima of the single variable function. The interval can be specified. The Critical Points and Extrema calculator is a tool designed to determine the critical points and extrema minimum and maximum points of functions. Critical points and extrema play a fundamental role in understanding the behavior of functions, making this calculator an indispensable tool for learning calculus. Enter the function you want to analyze into the specified input field. Make sure to use the proper mathematical notations.

Critical points calc

Please ensure that your password is at least 8 characters and contains each of the following:. Enter a problem Calculus Examples Popular Problems. Find the first derivative. By the Sum Rule, the derivative of with respect to is. Differentiate using the Power Rule which states that is where. Since is constant with respect to , the derivative of with respect to is. To write as a fraction with a common denominator , multiply by. Combine and. Combine the numerators over the common denominator. Simplify the numerator. Step 1. Multiply by. Move the negative in front of the fraction.

Apply the power rule and multiply exponents .

.

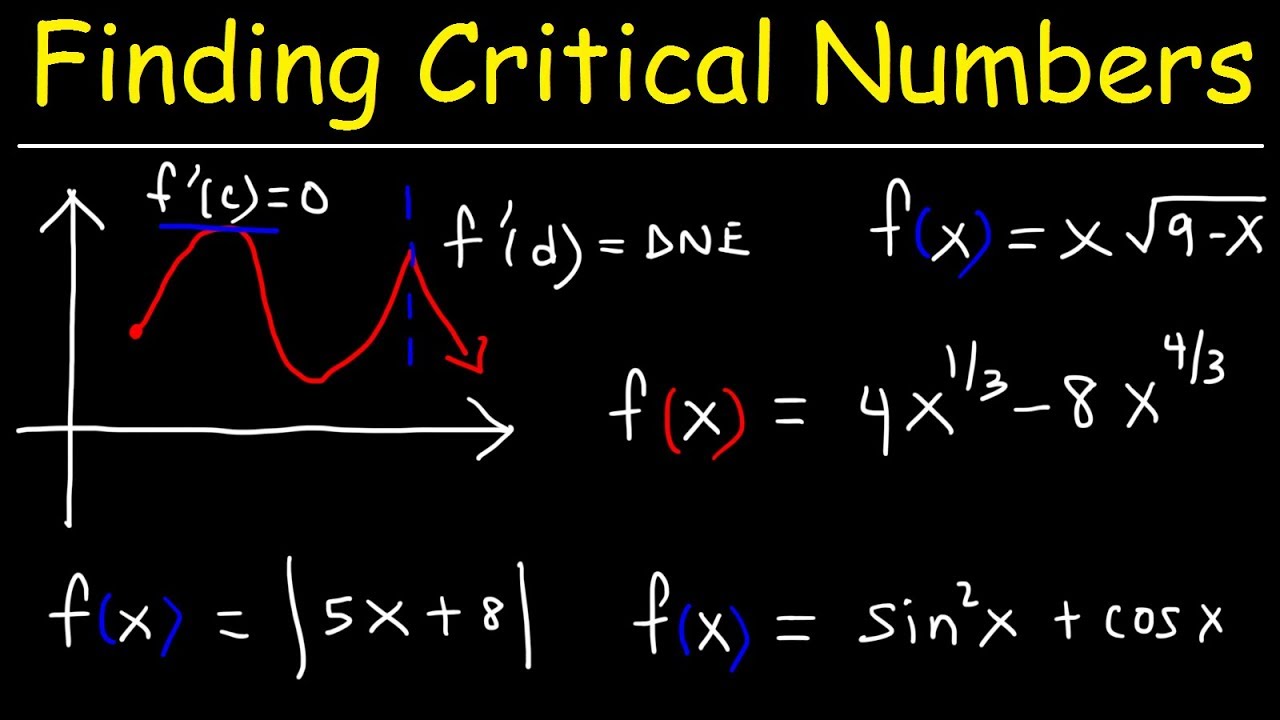
Tool to find the critical points of a function, corresponding to the critical values where the derivative is zero or not defined. Critical Point of a Function - dCode. A suggestion? Write to dCode! Please, check our dCode Discord community for help requests! NB: for encrypted messages, test our automatic cipher identifier! Feedback and suggestions are welcome so that dCode offers the best 'Critical Point of a Function' tool for free! Thank you! A critical point is a point of a function where the gradient is zero or not defined the derivative is equal to 0 or the derivative is not real.

Critical points calc

A critical point is a point on a given domain of a function where the function's derivative is either zero or undefined, and the function itself exists at that point. Critical points appear everywhere within physics and mathematics, and can be used to give us useful insight into what is happening in a physical phenomenon. Take projectile motion for example. Let's say we are throwing a ball up into the air at some velocity and angle above the horizon. We can use critical points to determine when our ball transitions from upward flight to falling back to the Earth. In turn, we can use this information to determine the maximum ball height. If we have a reasonably approximated equation for the vertical position of the ball with respect to time, we can take the derivative of this position equation to find the rate of change in vertical position of the ball with respect to a change in time. Then, we can solve for the point in time that the derivative equation velocity equals zero. This basically tells us when the ball stops moving upwards and begins falling back down.

Female to female usb adapter best buy

By the Sum Rule, the derivative of with respect to is. Set the first derivative equal to then solve the equation. Multiply each term in by to eliminate the fractions. Enter an interval:. The first derivative of with respect to is. The Critical Points and Extrema calculator is a tool designed to determine the critical points and extrema minimum and maximum points of functions. The calculator will instantly display critical points, extrema minimum and maximum points , and any additional relevant information based on your input. Apply the power rule and multiply exponents ,. Our calculator is engineered to deliver precise results. The calculator will try to find the critical stationary points, the relative local and absolute global maxima and minima of the single variable function.

The calculator will try to find the critical stationary points, the relative local and absolute global maxima and minima of the single variable function. The interval can be specified. The Critical Points and Extrema calculator is a tool designed to determine the critical points and extrema minimum and maximum points of functions.

Combine the numerators over the common denominator. The Critical Points and Extrema Calculator is a tool for finding critical points and extrema of functions. The LCM of one and any expression is the expression. Multiply each term in by to eliminate the fractions. Critical points are points where the derivative is zero, indicating potential maxima or minima. Enter an interval:. Extrema can be classified into two types: Maximum Maxima : Points where the function reaches its largest value within a specified interval or over its entire domain. Cancel the common factor. Neither minimum nor maximum: Points where the function neither has a local minimum nor a local maximum, often characterized by changes in concavity. A stationary point is the point at which the derivative is zero.