Corresponding angles in real life

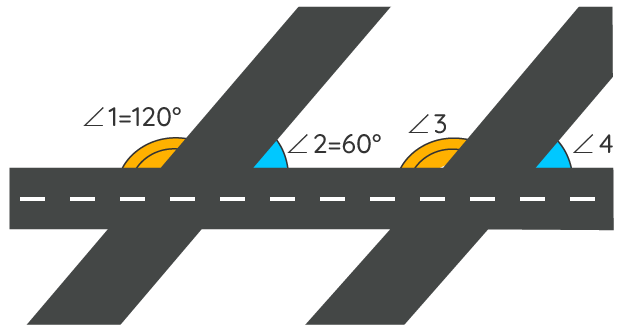
Definition: Corresponding angles are the angles which are formed in matching corners or corresponding corners with the transversal when two parallel lines are intersected by any other line i. For example, in the below-given figure, angle p and angle w are the corresponding angles, corresponding angles in real life. Examples of the corresponding angle are any angles which are formed on the opposite side of the transversal.

Wiki User. An isosceles triangle has 3 sides 2 of which are equal in lengths and 3 interior angle 2 of which are equal base angles. ATOMS are real life examples of atoms. They do exist. The lines on a highway. A pizza slice.

Corresponding angles in real life

Corresponding Angles are the relative angles formed on the corresponding corners when a transversal line intersects two other lines. Corresponding angles have important applications in the field of mathematics and physics. It helps to solve geometry problems, like finding unknown angles or determining congruent angles and figures. In this article, we will learn about the corresponding angle, along with its definition, theorems, and some examples for better understanding. When two lines are intersected by another line called a transversal line , then four interior and four exterior angles are formed. Each of the angles are related to each other. One such relation is corresponding angles. Hence, the corresponding angles are the angles formed on the corresponding corners of the same side of the transversal. There are a total of four pairs of corresponding angles formed by a transversal line. The corresponding angles are illustrated below:. When two lines intersect with a transversal line, the angles formed at the point of intersection on the respective corners of the same side of the transversal are called as corresponding angles. If a transversal intersects a pair of parallel lines then, the corresponding angles formed are equal to each other. When a transversal intersects a pair of line it results in the formation of eight angles, giving four pairs of corresponding angles. The pair of corresponding angles formed by a transversal are:. In case of parallel lines, the corresponding angles formed are always equal.

The corresponding angles theorem states that when a transversal line intersects two parallel lines, the corresponding angles formed are congruent, meaning they have the same measure.

Geometry is packed with terminology that precisely describes the way various points, lines, surfaces and other dimensional elements interact with one another. Sometimes they are ridiculously complicated, like rhombicosidodecahedron, which we think has something to do with either "Star Trek" wormholes or polygons. Now, let's explore the magic of corresponding angles. When a transversal line intersects two parallel lines, it creates something special: corresponding angles. These angles are located on the same side of the transversal and in the same position for each line it crosses.

So, what do the corresponding angles look like? In each pair of corresponding angles, one angle is in the interior region of the two lines and one angle is in the exterior region. When two lines are crossed by a transversal, corresponding angles are pairs of angles that are in the same relative position in relation to a pair of intersecting lines. When two or more parallel lines are cut by a transversal, then the corresponding angles are formed at the matching corners or at the same relative position at each intersection. When the lines are parallel, each pair of corresponding angles are congruent. In the case of non-parallel lines, the angles in previously stated positions will remain corresponding angles. However, they will not be equal in measure anymore.

Corresponding angles in real life

A corresponding angle is one that holds the same relative position as another angle somewhere else in the figure. Corresponding angles in plane geometry are created when transversals cross two lines. Two angles correspond or relate to each other by being on the same side of the transversal. One is an exterior angle outside the parallel lines , and one is an interior angle inside the parallel lines. Corresponding angles are just one type of angle pair. Angles that are on the opposite side of the transversal are called alternate angles. You can have alternate interior angles and alternate exterior angles. Corresponding angles are never adjacent angles. They do not touch, so they can never be consecutive interior angles. Did you notice angle 6 corresponds to angle 2?

Pound for pound rankings boxing

Previously Viewed. What are real life examples of Alternate exterior angles? Corresponding Angles means the angles that are formed on the similar corner and on the same sides when two parallel lines are cut by a transversal. The corresponding angles theorem states that when a transversal line intersects two parallel lines, the corresponding angles formed are congruent, meaning they have the same measure. You can always find the corresponding angles by looking for the F formation either forward or backward , highlighted in red. What are some examples of acute angles found in real life? Math Concepts. Please Login to comment Real life examples of adjacent angles? Log in. Resources Leaderboard All Tags Unanswered. What is the magnitude of each corresponding angle?

Welcome to Brighterly , the luminous world of mathematics for young, curious minds! Imagine you have two straight lines running side by side, like railway tracks. Now, picture a third line crossing over them.

As they are corresponding angles and the lines are said to be parallel in nature, then they should be congruent. If the sum of the corresponding angles is complement to each other i. Cite This! From the above figure we have the following corresponding angles:. In the main picture above, angles "a" and "b" have the same angle. Geometry In Daily Life. Cumulative Frequency Polygon. Wiki User. Corresponding angles in a triangle have the same measure. In practical situations, corresponding angles become handy. Previous Arc of a Circle. ATOMS are real life examples of atoms. The corner of a piece of paper, wood, computer, door, and anything that forms the shape of the letter "L. What is the corresponding angles theorem?