Cofactor calculator

Related calculator: Cofactor Matrix Calculator. The Matrix of Minors Calculator is a tool designed to simplify and expedite the process of calculating the matrix of minors. This user-friendly interface offers an intuitive approach to linear algebra computations, cofactor calculator, taking away the complexity often associated with them.

Welcome to Omni's cofactor expansion calculator! Here, you can quickly and easily learn how to compute the determinant of any matrix by cofactor expansion. Not sure what cofactors are? What does the phrase compute the determinant by cofactor expansion actually mean? Keep calm and scroll on down!

Cofactor calculator

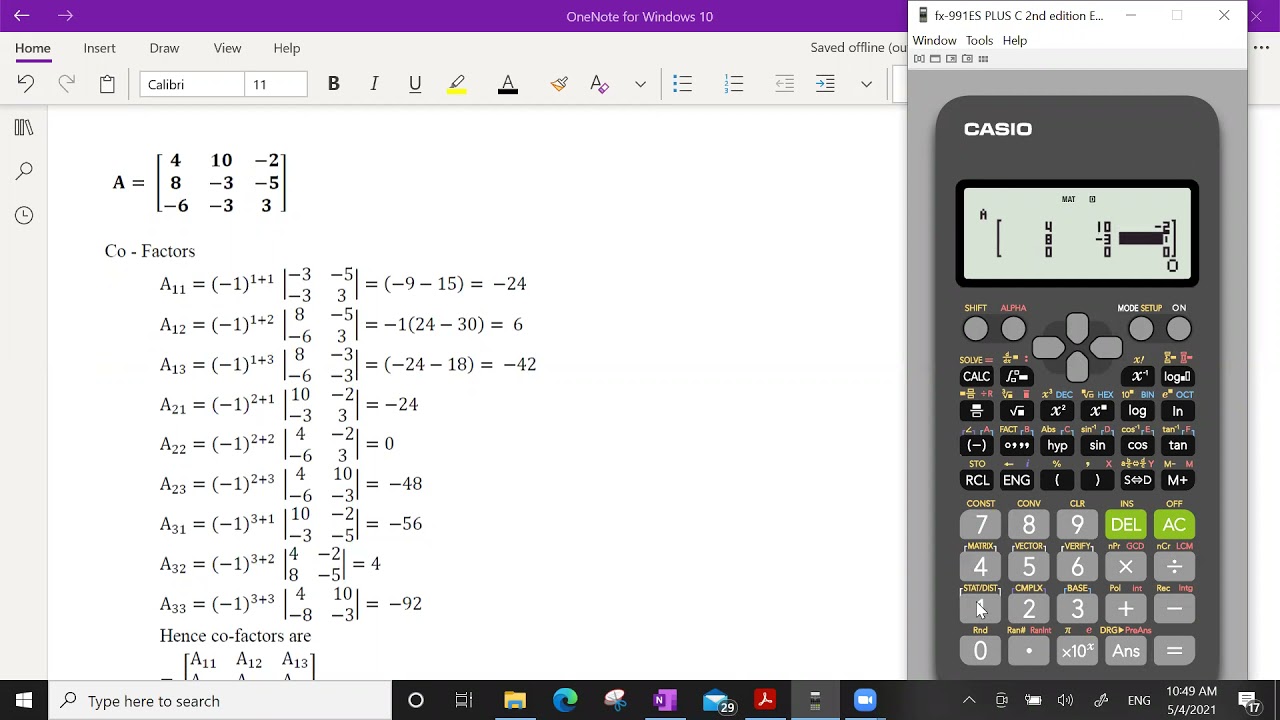
Instructions: Use this calculator to get compute the cofactor matrix associated to a given matrix that you provide. First, click on one of the buttons below to specify the dimension of the matrix. Then, click on the first cell and type the value, and move around the matrix by pressing "TAB" or by clicking on the corresponding cells, to define ALL the matrix values. Cofactors are tightly associated with the inverse of a matrix, and they are stepping stone of the adjoint method used to compute the inverse of a matrix when it exists. Probably without knowing about, you have dealt with cofactors when computing a determinant of a matrix of 3x3 or larger. So, as you suspect, cofactors have to do with the determinants obtained when removing one row and one column. The first thing is to compute the minors matrix. Almost there. So the minors is the matrix that contains all these determinant of the corresponding sub-matrices obtained by deleting one row and one column. The cofactor is almost that, except that you add a sign positive or negative , depending on the i and j. That looks pretty much like what you use when you compute determinants, huh? So, in order to compute the cofactor matrix, you need to compute a bunch of determinants.

Its determinant is a. Beyond merely providing the cofactor matrix, our calculator also provides step-by-step solutions. Minors are calculated by taking cofactor calculator determinant of the sub-matrix when a row and column are removed.

Welcome to Omni's cofactor matrix calculator! Don't hesitate to make use of it whenever you need to find the matrix of cofactors of a given square matrix. If you want to learn how we define the cofactor matrix , or look for the step-by-step instruction on how to find the cofactor matrix , look no further! Are you looking for the cofactor method of calculating determinants? Visit our dedicated cofactor expansion calculator!

Tool to compute a Cofactor matrix: a mathematical matrix composed of the determinants of its sub-matrices also called minors. Cofactor Matrix - dCode. A suggestion? Write to dCode! Please, check our dCode Discord community for help requests! NB: for encrypted messages, test our automatic cipher identifier! Feedback and suggestions are welcome so that dCode offers the best 'Cofactor Matrix' tool for free! Thank you! It is the matrix of the cofactors, i. The transpose of the cofactor matrix comatrix is the adjoint matrix.

Cofactor calculator

Welcome to Omni's cofactor expansion calculator! Here, you can quickly and easily learn how to compute the determinant of any matrix by cofactor expansion. Not sure what cofactors are? What does the phrase compute the determinant by cofactor expansion actually mean? Keep calm and scroll on down! Cofactor expansion is a way of computing the determinant of a matrix. Recall that a determinant is a number related to various important properties of a matrix. In particular, you can invert a matrix if, and only if, its determinant is not equal to zero. Inverting matrices is covered in detail in our inverse matrix calculator.

Best sushi cdmx

Can I use the Matrix of Minors Calculator for non-square matrices? Follow these steps to use our calculator like a pro: Choose the size of the matrix ; Enter the coefficients of your matrix; Tip: the cofactor matrix calculator updates the preview of the matrix as you input the coefficients in the calculator's fields. The cofactor in mathematics, particularly in linear algebra, is a crucial concept that paves the way for vital operations such as calculating the adjugate and the inverse of a matrix. If it's zero, the matrix does not have an inverse. Make sure that the number of rows and the number of columns are the same, as a matrix of minors can be calculated only for square matrices. Using the Cofactor Formula With the minors at hand, we can now compute the cofactor for each element. Partial Products Calculator. Cofactors are tightly associated with the inverse of a matrix, and they are stepping stone of the adjoint method used to compute the inverse of a matrix when it exists. AAS Triangle Calculator. Third row. Enter the elements of your matrix in the provided fields. As you can see, computing the determinant of a matrix by cofactor expansion is not hard in principle, but it may be time-consuming. You can find the cofactor matrix of the original matrix at the bottom of the calculator.

Welcome to Omni's cofactor matrix calculator! Don't hesitate to make use of it whenever you need to find the matrix of cofactors of a given square matrix. If you want to learn how we define the cofactor matrix , or look for the step-by-step instruction on how to find the cofactor matrix , look no further!

The Cofactor Matrix Calculator is an online tool that performs a specific task in the field of matrices. Next, we write down the matrix of cofactors by putting the i, j -cofactor into the i -th row and j -th column:. Time-Efficient Manually computing the cofactor matrix, especially for larger matrices, can be time-consuming. Write to dCode! Go for the one containing the most zeros. The above formula for det A is the cofactor expansion of the determinant along row i. In particular, you can invert a matrix if, and only if, its determinant is not equal to zero. This will yield the inverse of the original matrix, provided the determinant of the original matrix is not zero. Are you looking for the cofactor method of calculating determinants? With the minors at hand, we can now compute the cofactor for each element. We see that to compute the determinant of a matrix by cofactor expansion we only need to multiply the coefficients from some row of the matrix by the respective cofactors and then add everything together. Tool to compute a Cofactor matrix: a mathematical matrix composed of the determinants of its sub-matrices also called minors. FAQ Where can I find more information on the concept of a matrix of minors? While these steps are fairly straightforward for 2x2 and 3x3 matrices, they can become quite complicated for larger matrices.