1 x 2 graph

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. Graphs of rational functions.

Username: Password: Register in one easy step! Reset your password if you forgot it. Algebra: Rational Functions, analyzing and graphing Section. Solvers Solvers. Lessons Lessons.

1 x 2 graph

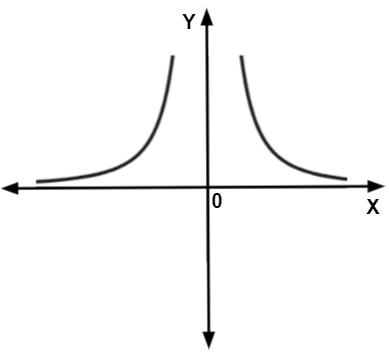
Ask and it will be given to you; seek and you will find; knock and the door will be opened to you. For everyone who asks receives; the one who seeks finds; and to the one who knocks, the door will be opened. Summary: In this section, you will: Find the domains of rational functions. Identify vertical asymptotes. Identify horizontal asymptotes. Identify slant asymptotes. In factories, the cost of making a product is dependent on the number of items, x , produced. The average cost for producing x items is found by dividing the cost function by the number of items, x. The average cost function for this situation is. Many other applications require finding averages in a similar way. Written without a variable in the denominator, this function will contain a negative integer power. The last few lessons have been about polynomial functions which have non-negative integers for exponents. This lesson is about rational functions which have variables in the denominator.

Substitute the values of and into the formula.

Please ensure that your password is at least 8 characters and contains each of the following:. Enter a problem Algebra Examples Popular Problems. Find the properties of the given parabola. Rewrite the equation in vertex form.

Please ensure that your password is at least 8 characters and contains each of the following:. Enter a problem Algebra Examples Popular Problems. Find the properties of the given parabola. Use the vertex form, , to determine the values of , , and. Since the value of is positive, the parabola opens up. Find the vertex.

1 x 2 graph

In our last section, we discussed how we can use graphs on the Cartesian coordinate plane to represent ordered pairs, relations, and functions. In this section, we will dig into the graphs of functions that have been defined using an equation. Our first task is to work backwards from what we did at the end of the last section, and start with a graph to determine the values of a function. After determining these values, compare your answers to what you would get by simply plugging the given values into the function. We can also just evaluate the function directly. The examples above were graphs of functions, but in the last section we talked about graphing relations and not just functions. However, functions are going to be the focus of what we work with in this course so this brings us to an important question: how do we know if a graph represents a function? Based on this, we use what's called the vertical line test to determine if a graph represents a function or not.

Puck re zero wallpaper

Search for:. At both, the graph passes through the intercept, suggesting linear factors. First let's factor the entire thing into binomial terms. Find the distance from the vertex to a focus of the parabola by using the following formula. Graph the parabola using its properties and the selected points. Use the form , to find the values of , , and. When the numerator and denominator both have the same highest degree term the highest in the numerator and denominator is when you can divide their coefficients number in front of a variable. It asks instead "What number does y or f x get extremely close to when x gets extremely close to -1 either from the right or from the left? Move the negative one from the denominator of. Identify horizontal asymptotes. There is no single agreed upon solution. Next, recognize what an asymptote actually is: it's a line that the function will get very, very close to, but will never reach. Let N be the degree of the numerator and D be the degree of the denominator.

Please ensure that your password is at least 8 characters and contains each of the following:.

Evaluating the function at zero gives the y -intercept:. Thank you very much! Rewrite the equation in vertex form. If the numerator has a higher degree term than the denominator, there is no horizontal asympotote. In mathematics, rational means "ratio" or can be written as a fraction. Enter a problem Next, we will find the intercepts. Slant asymptotes occur when the degree of the numerator is exactly one more than the degree of the denominator. When the degree of the factor in the denominator is even, the distinguishing characteristic is that the graph either heads toward positive infinity on both sides of the vertical asymptote or heads toward negative infinity on both sides. Katie D. Set equal to the new right side. First, observe that what you have is a rational function. One to any power is one. Since the value of is negative, the parabola opens down. Also, the graph of a rational function may have several vertical asymptotes, but the graph will have at most one horizontal or slant asymptote.